Fluctuations and randomness create the waves in which we live.
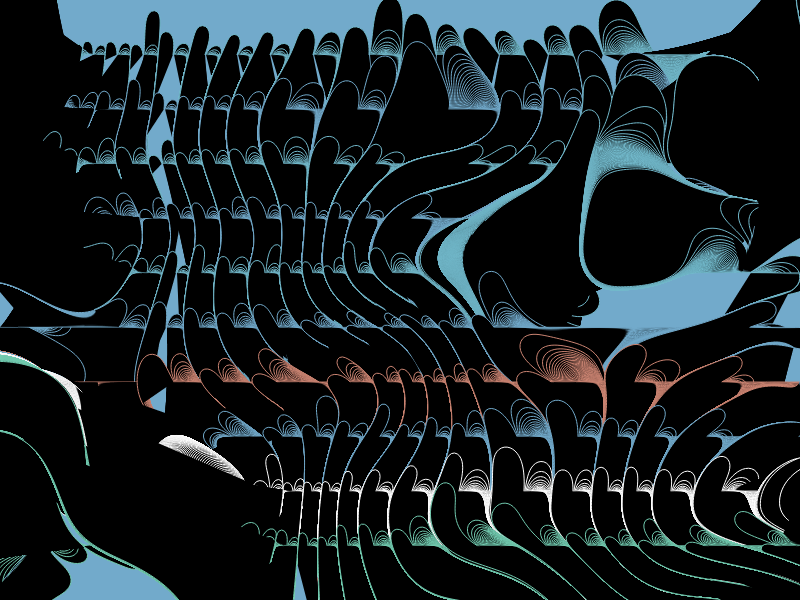
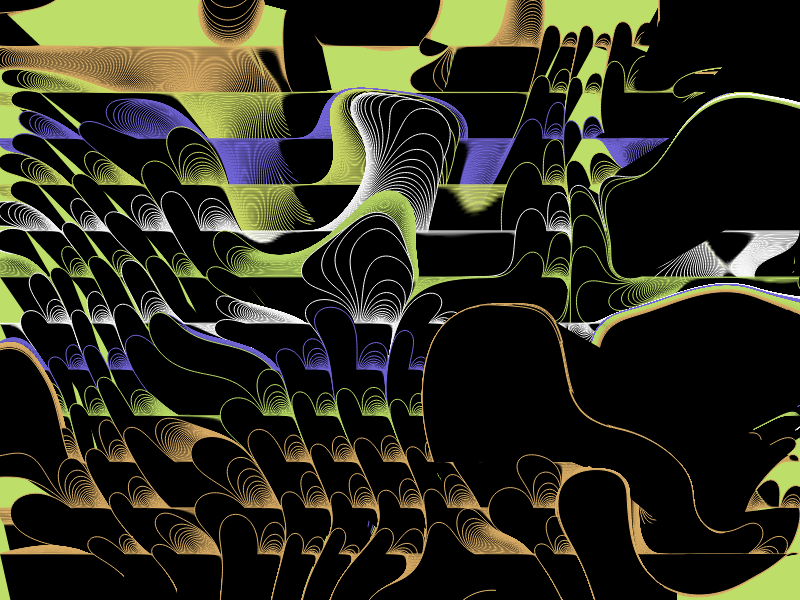
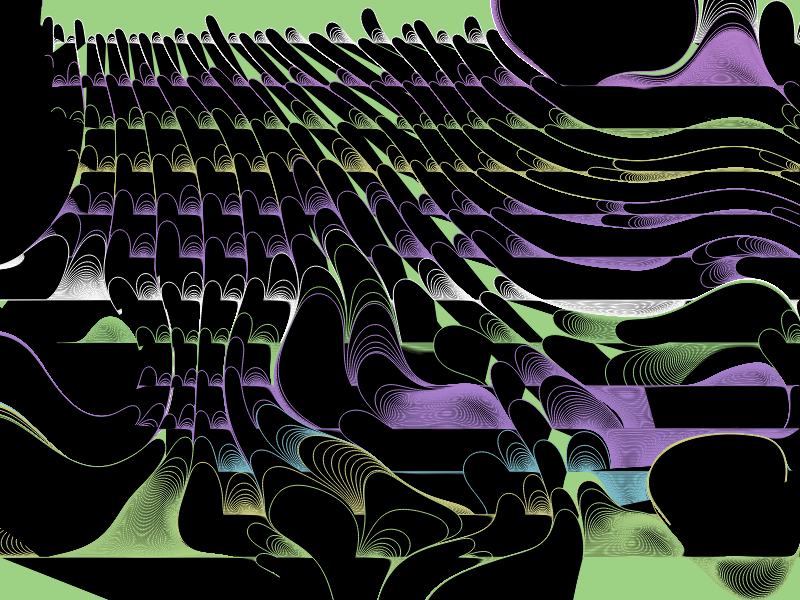
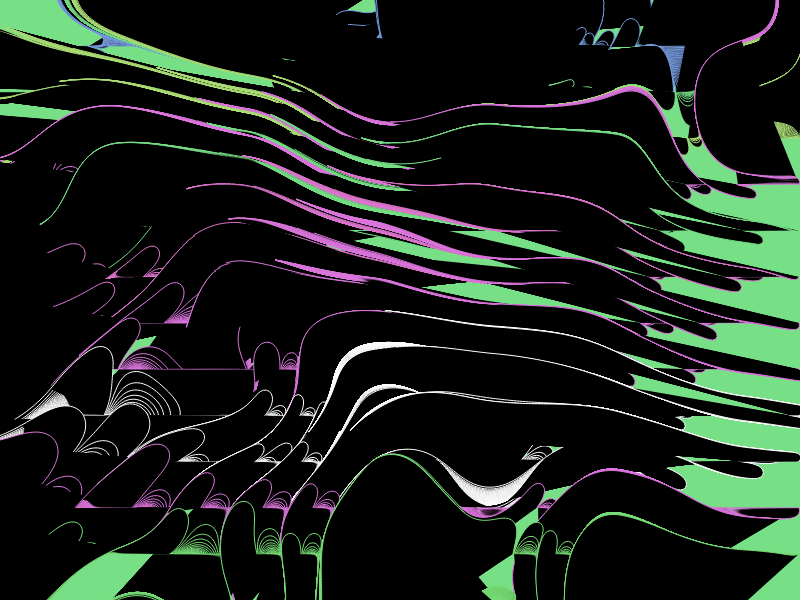
Second and last piece in "oscillation" theme.

----- Generative art as a discrete mathematics lesson -------
- What is a Graph?
A graph is a set of points, called nodes, connected by lines, called edges. Imagine a network of cities (nodes) connected by roads (edges).

- What is a Loop?
A loop in a graph is a path that starts at one node, travels through a series of edges, and returns to the same node. Think of walking in a circle starting and ending at your home.

-Loops in Graph Theory
There are two main types of loops:
.Self-loop: A node that connects back to itself.
.Cycle: A sequence of nodes where you start and end at the same node without retracing any edges.

Loops in GA
In generative art, loops can create patterns and structures. By using algorithms that generate loops, artists can create intricate designs that repeat and evolve. These loops can represent repeating themes, movements, or shapes, adding depth and complexity to the artwork.