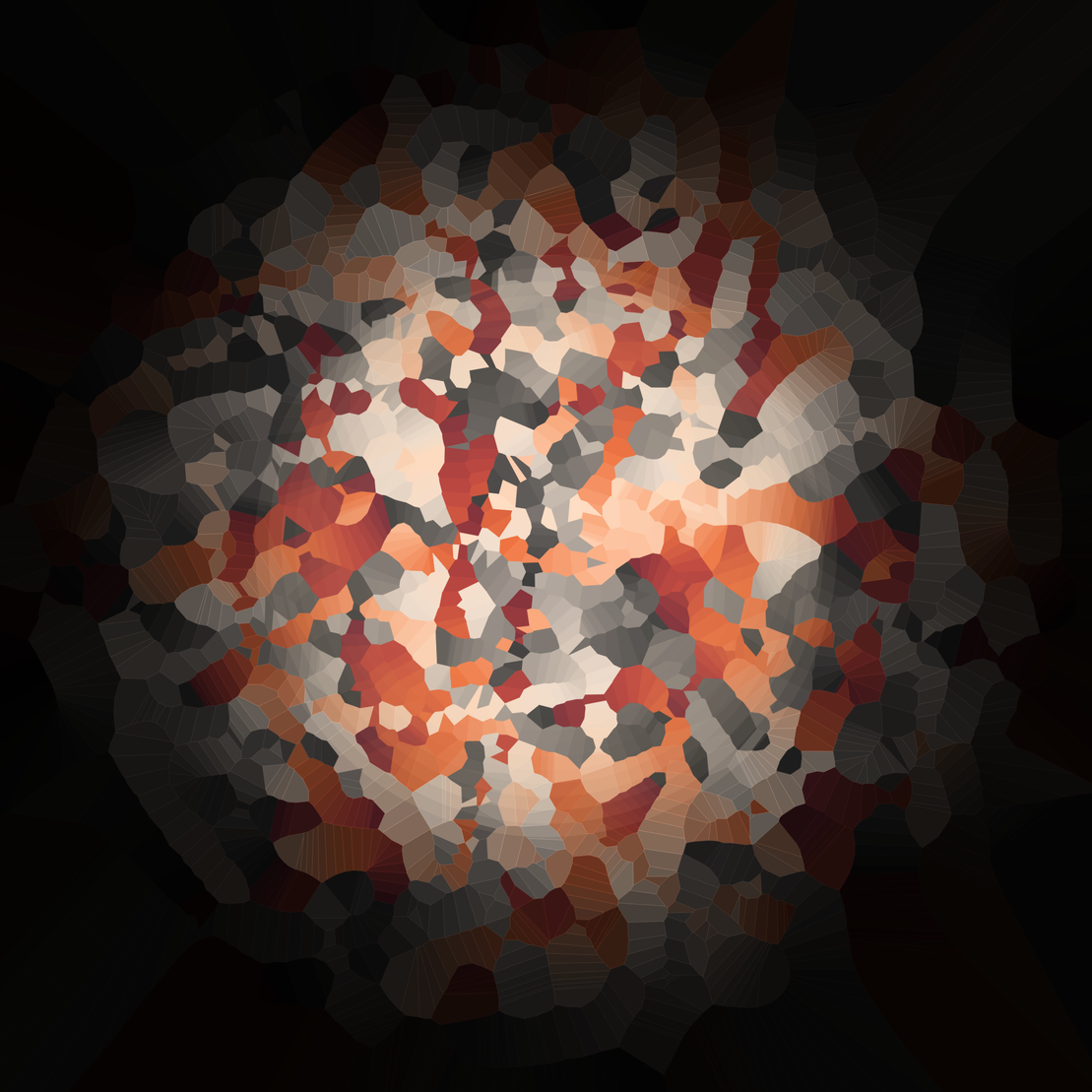
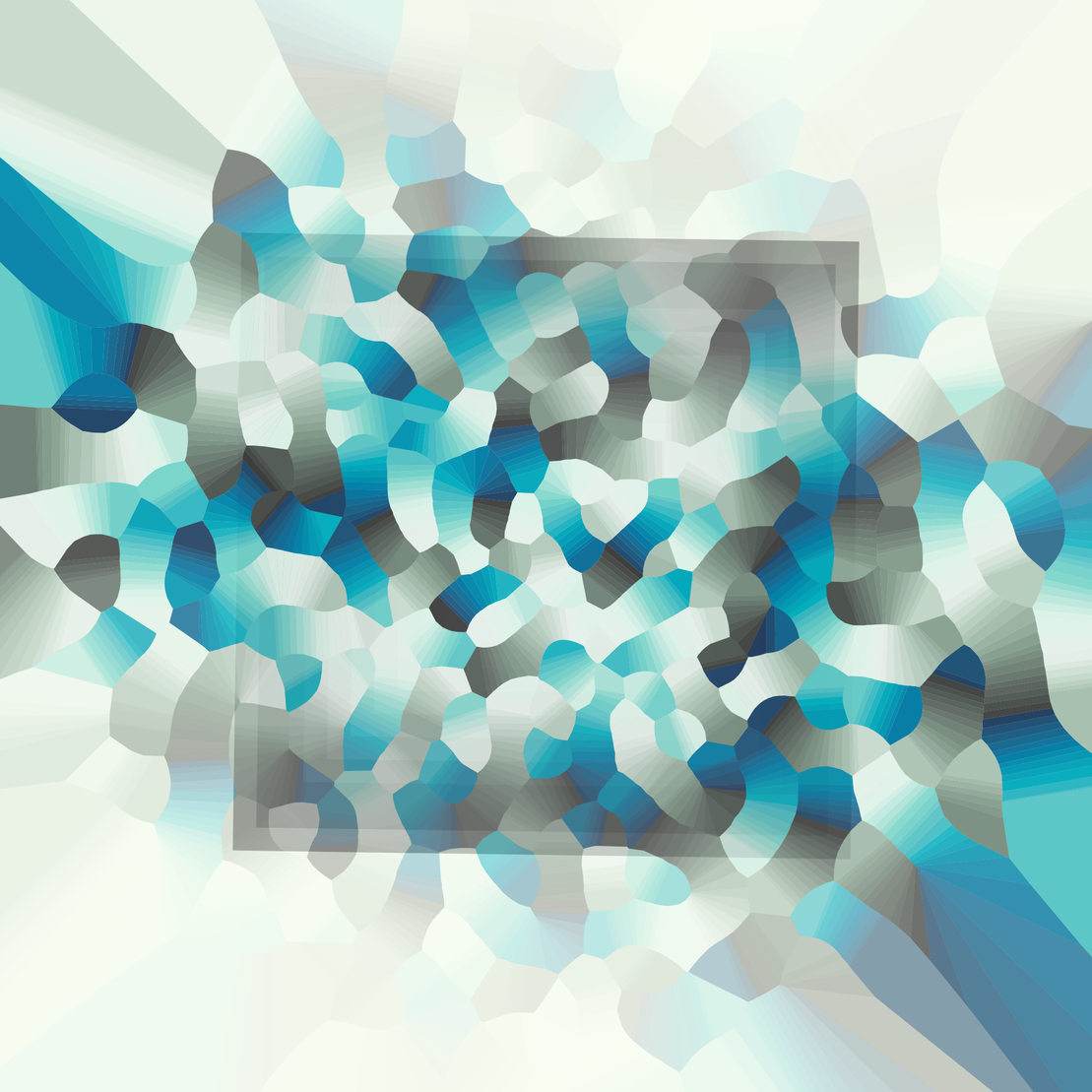
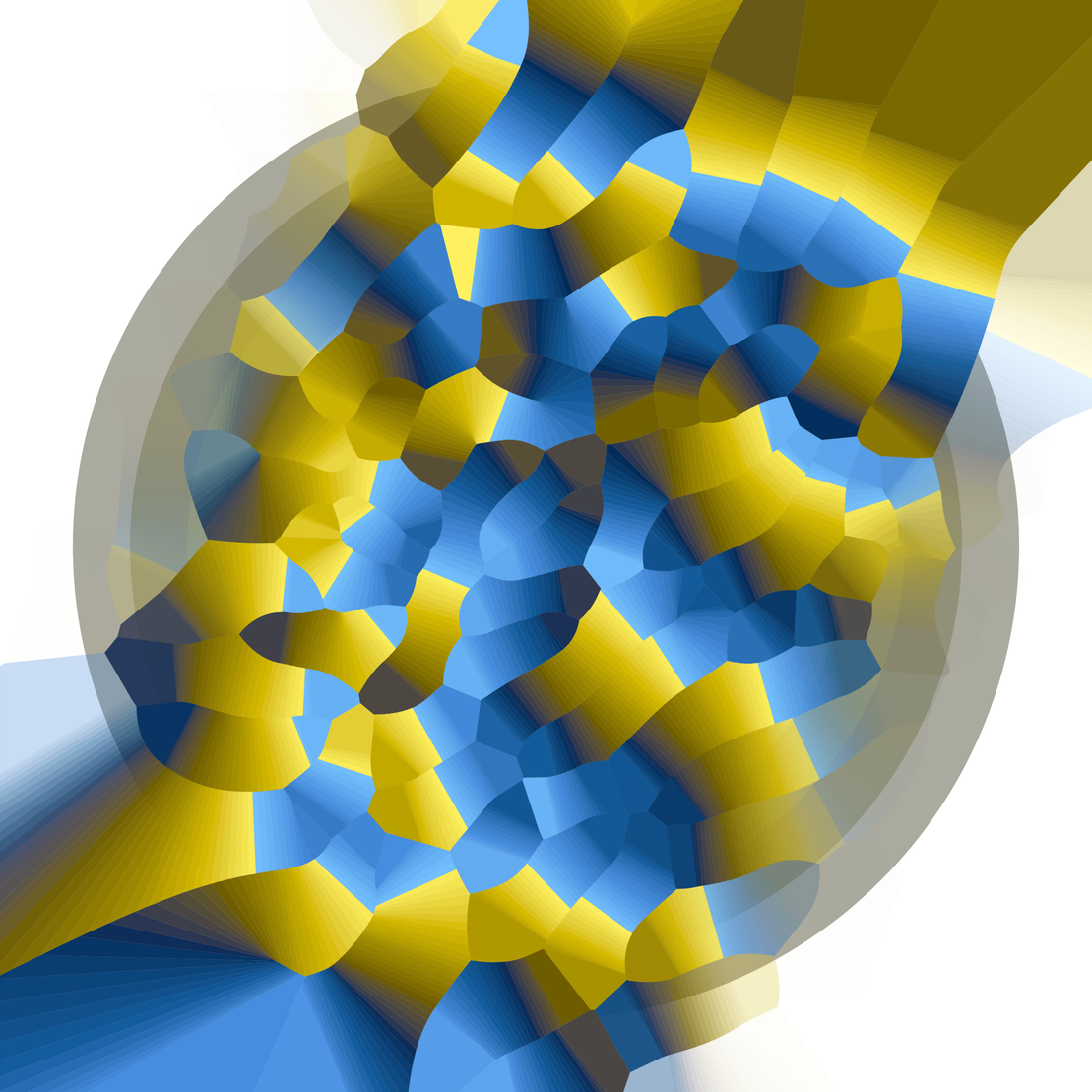
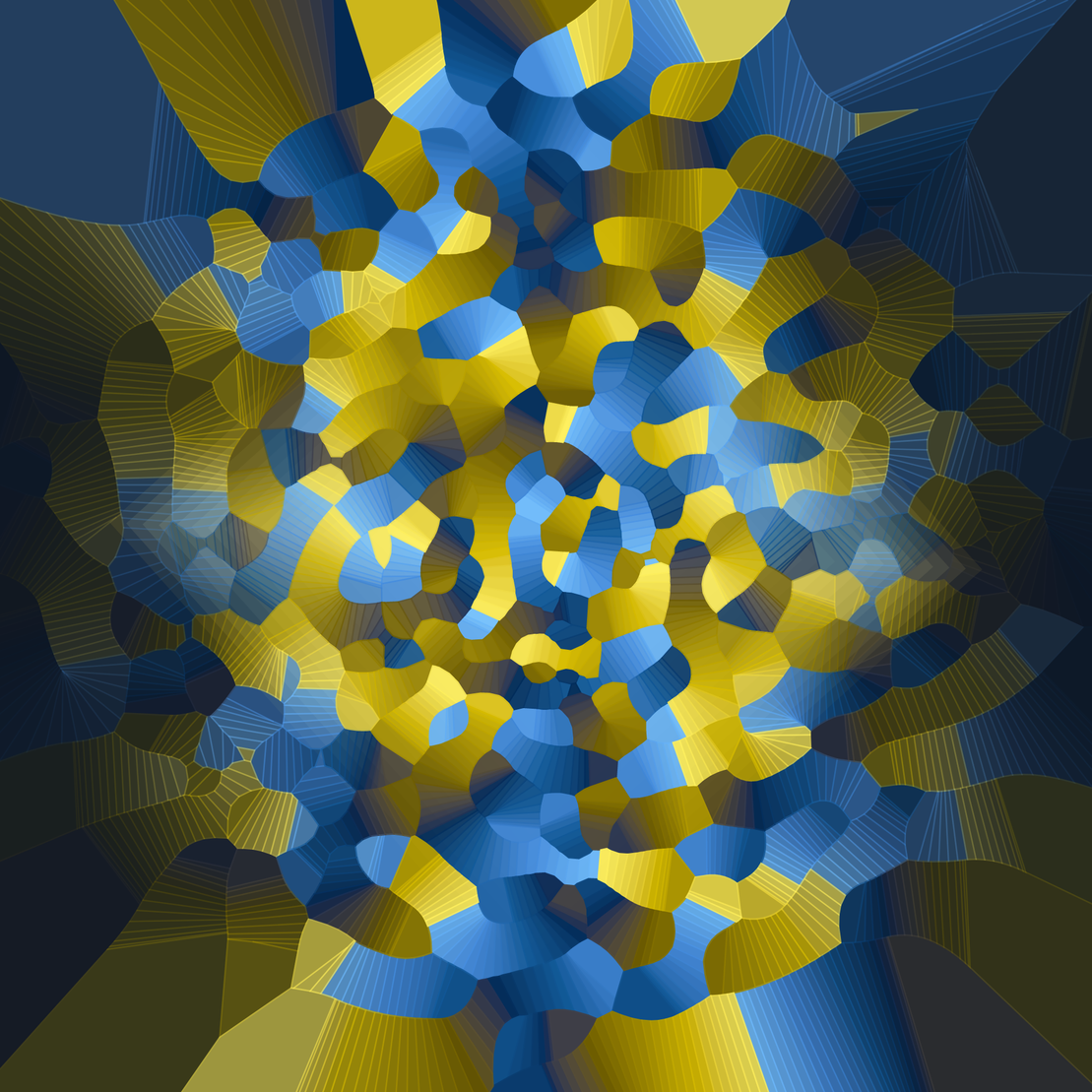
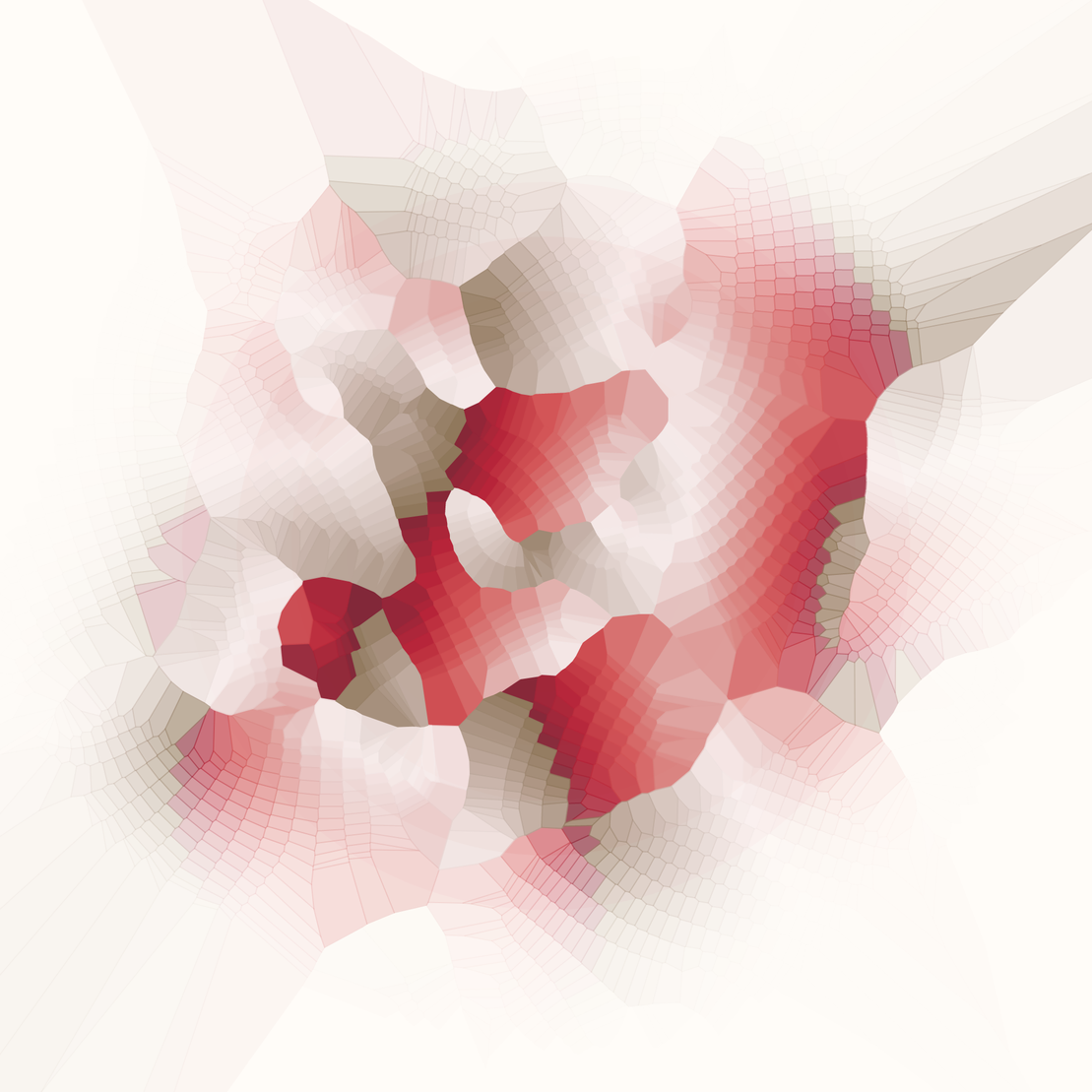
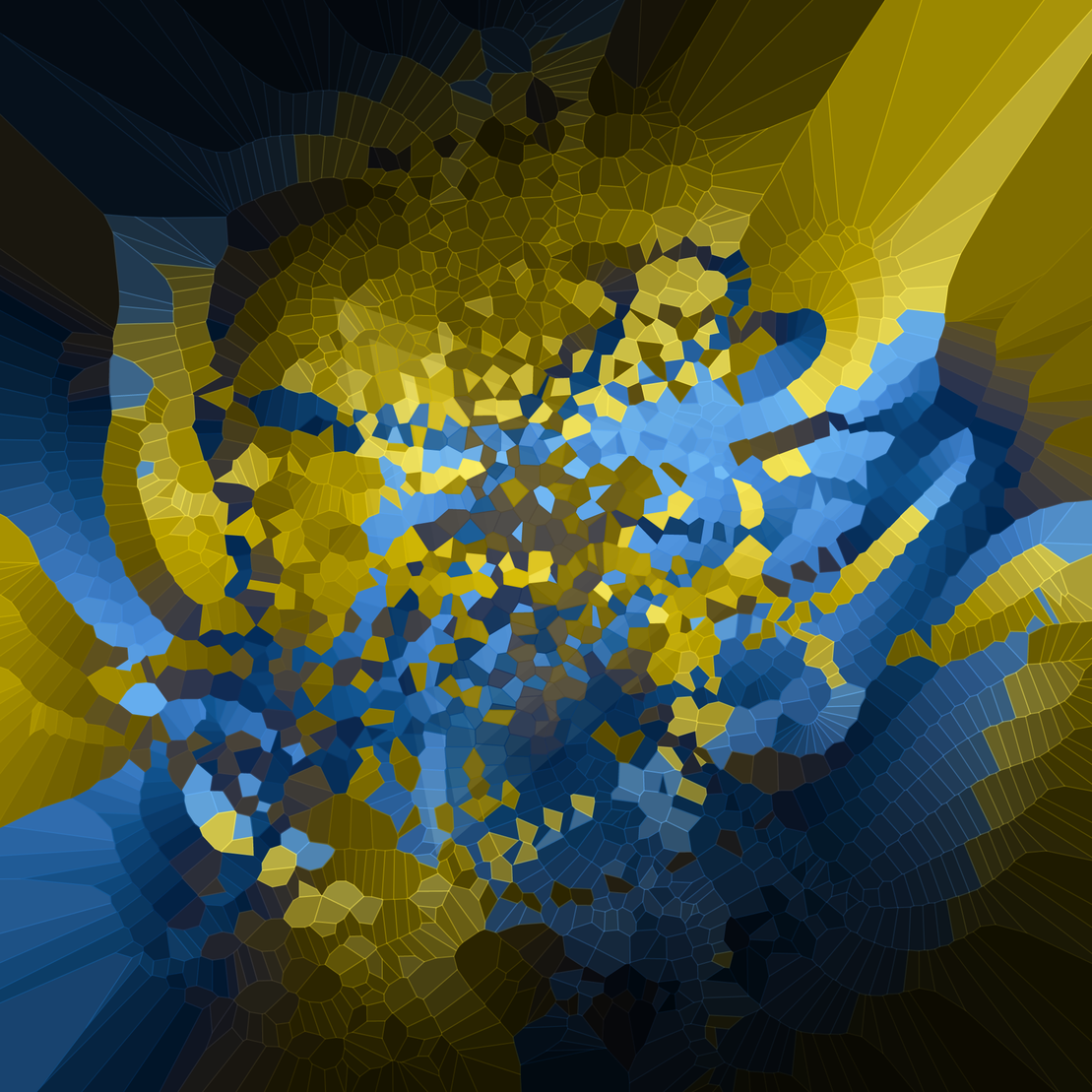
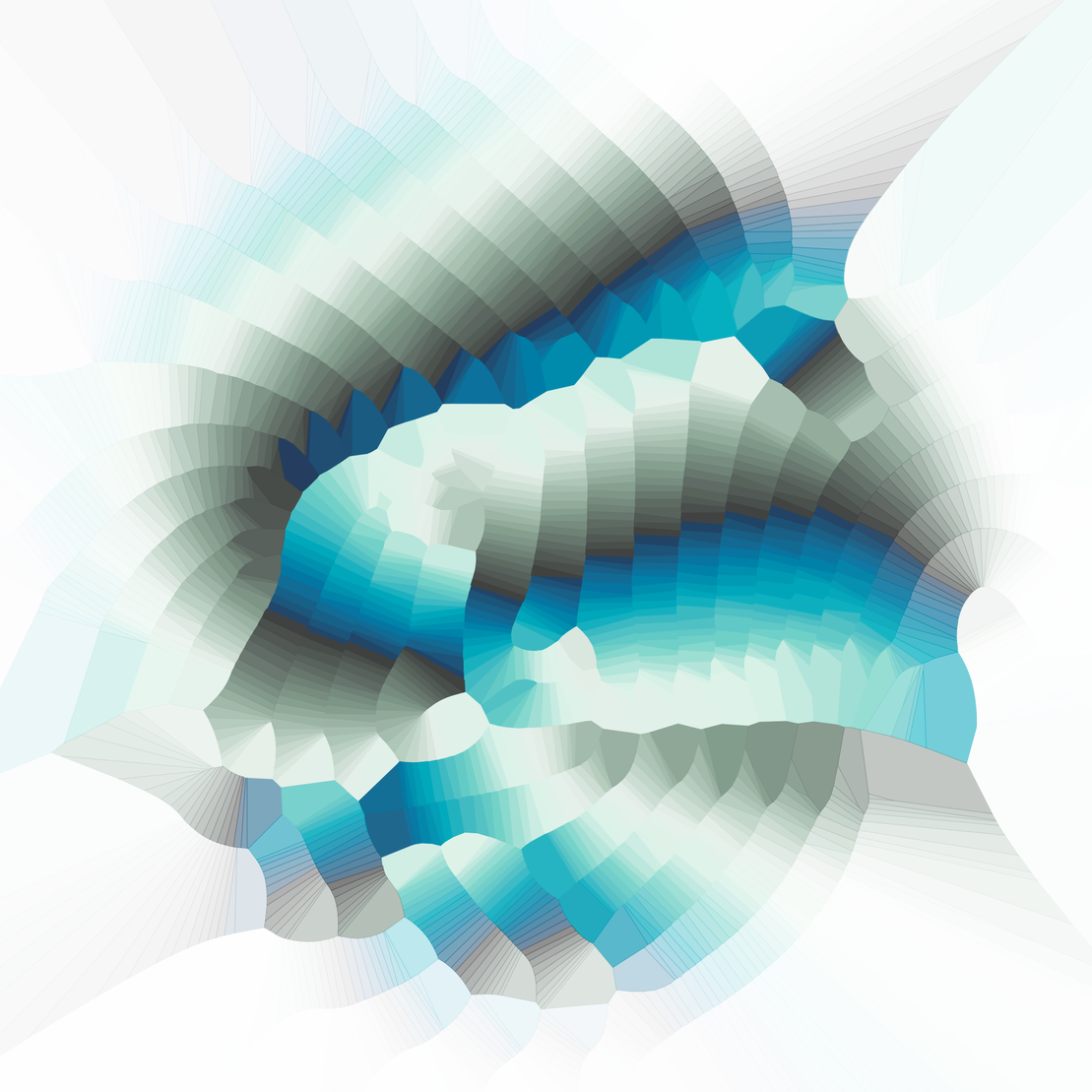
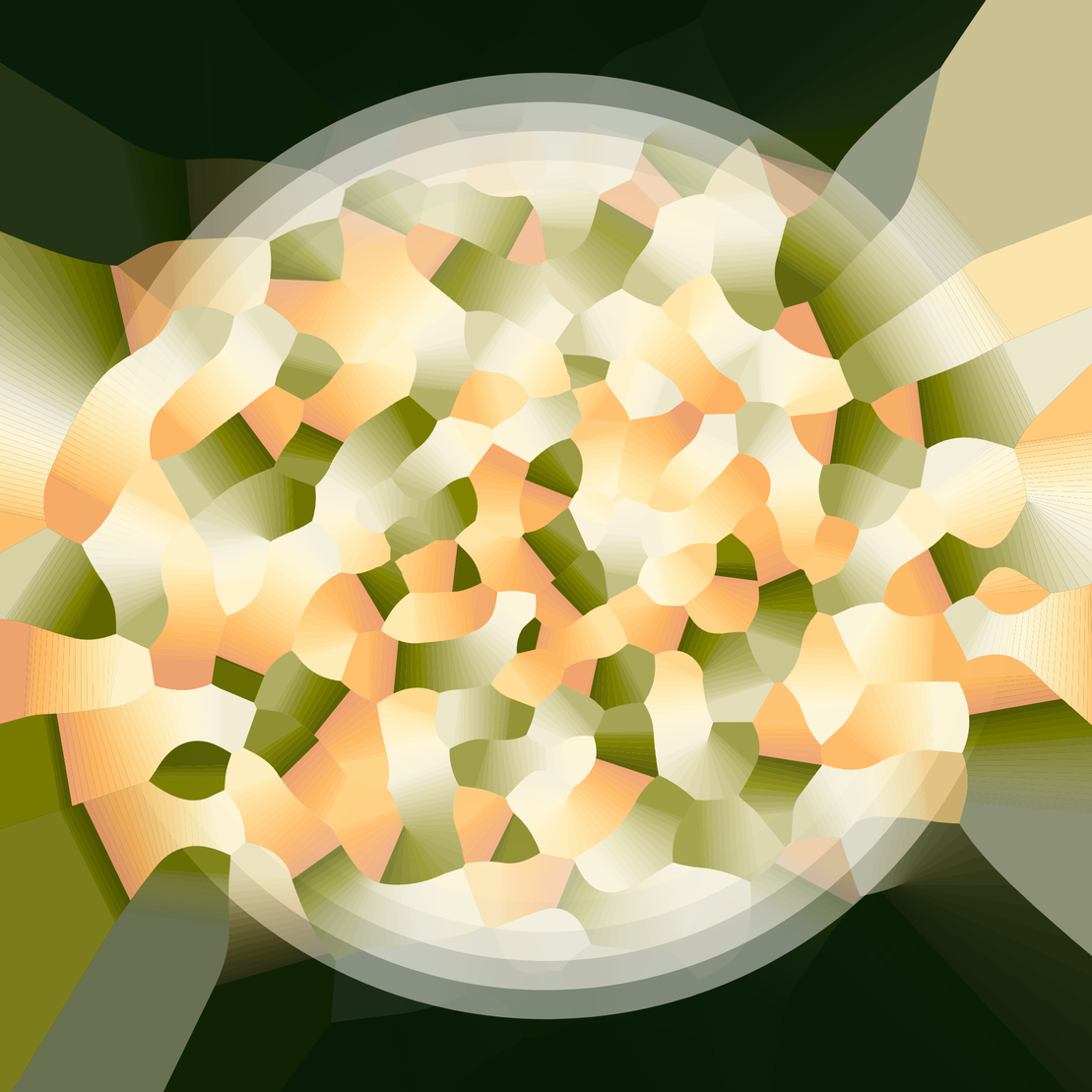
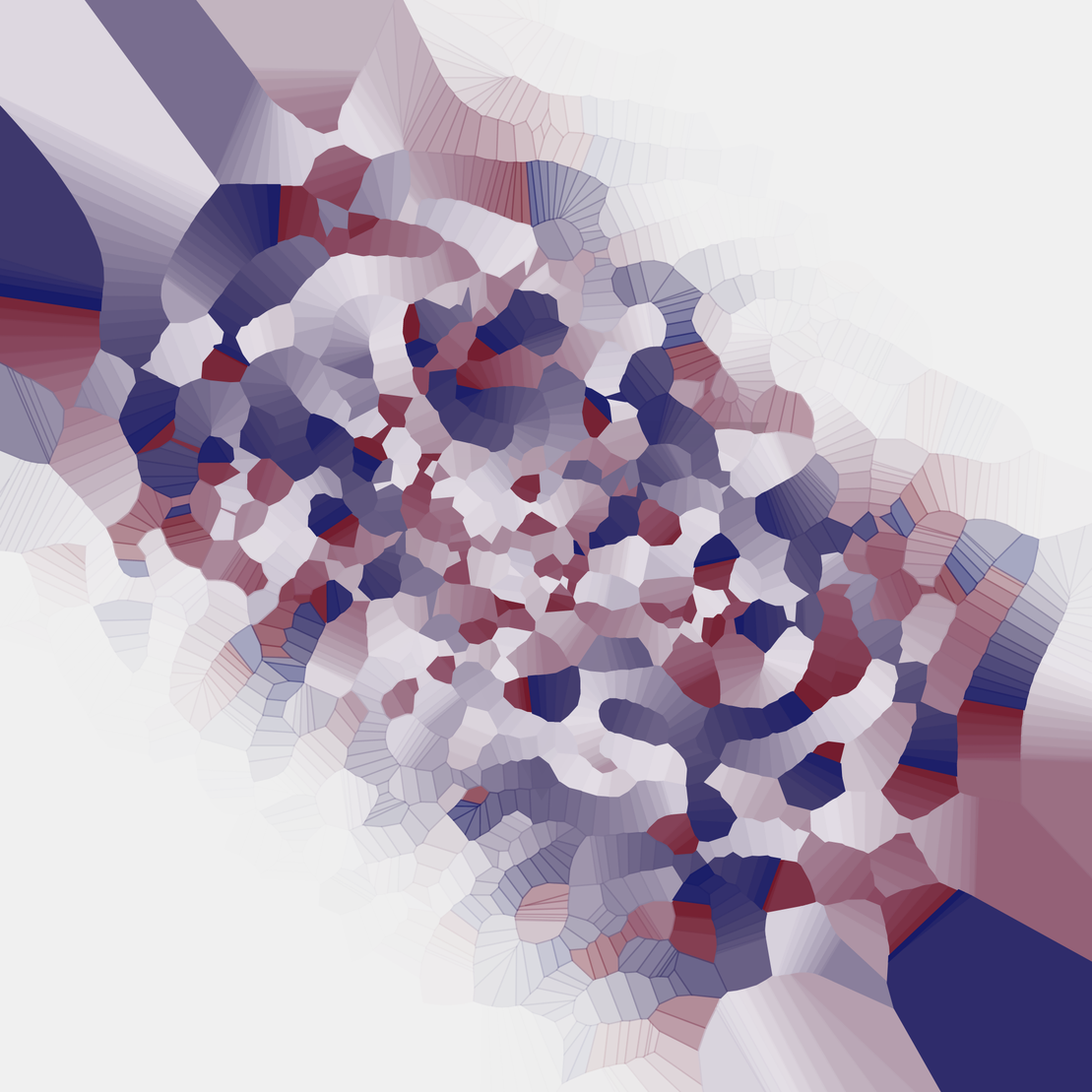
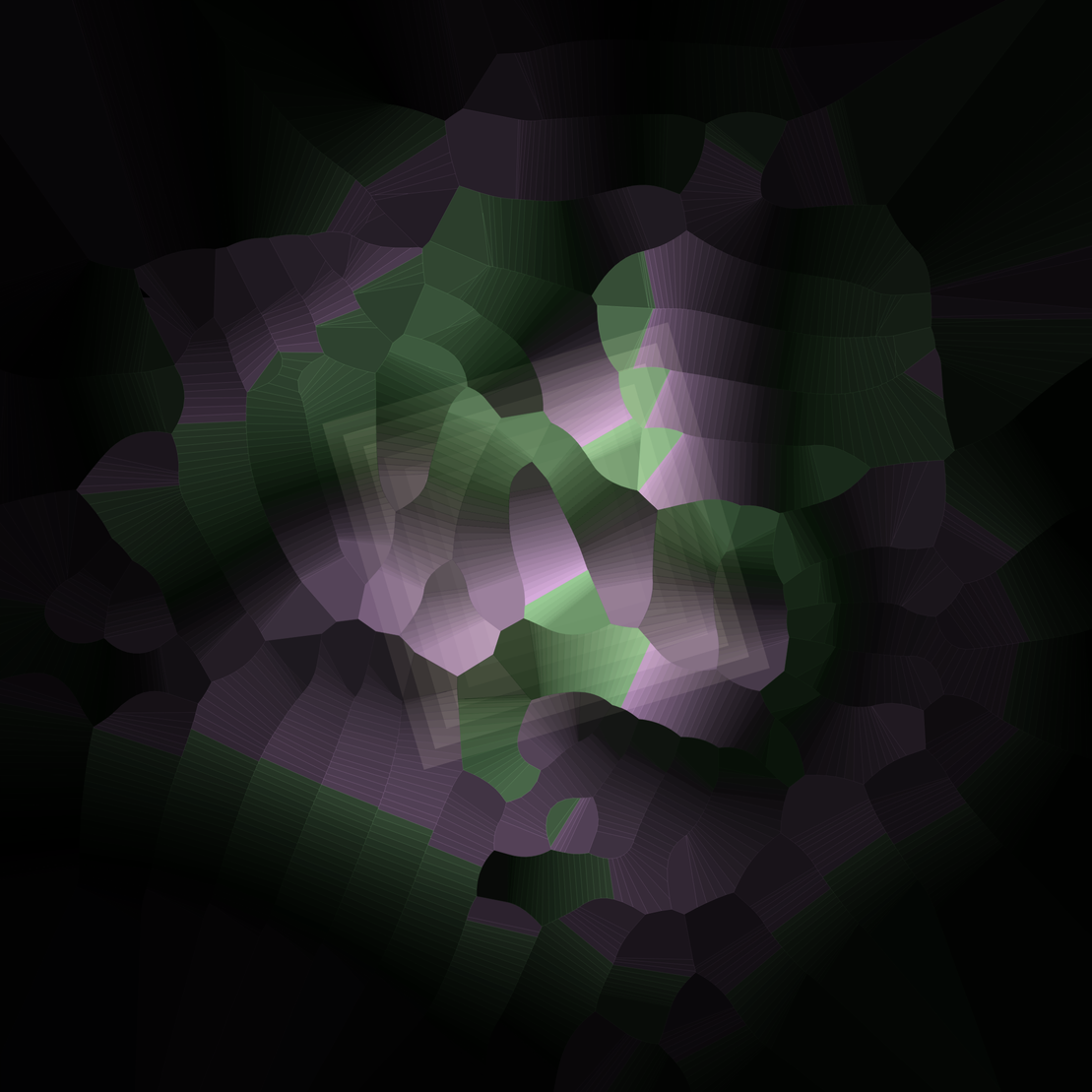
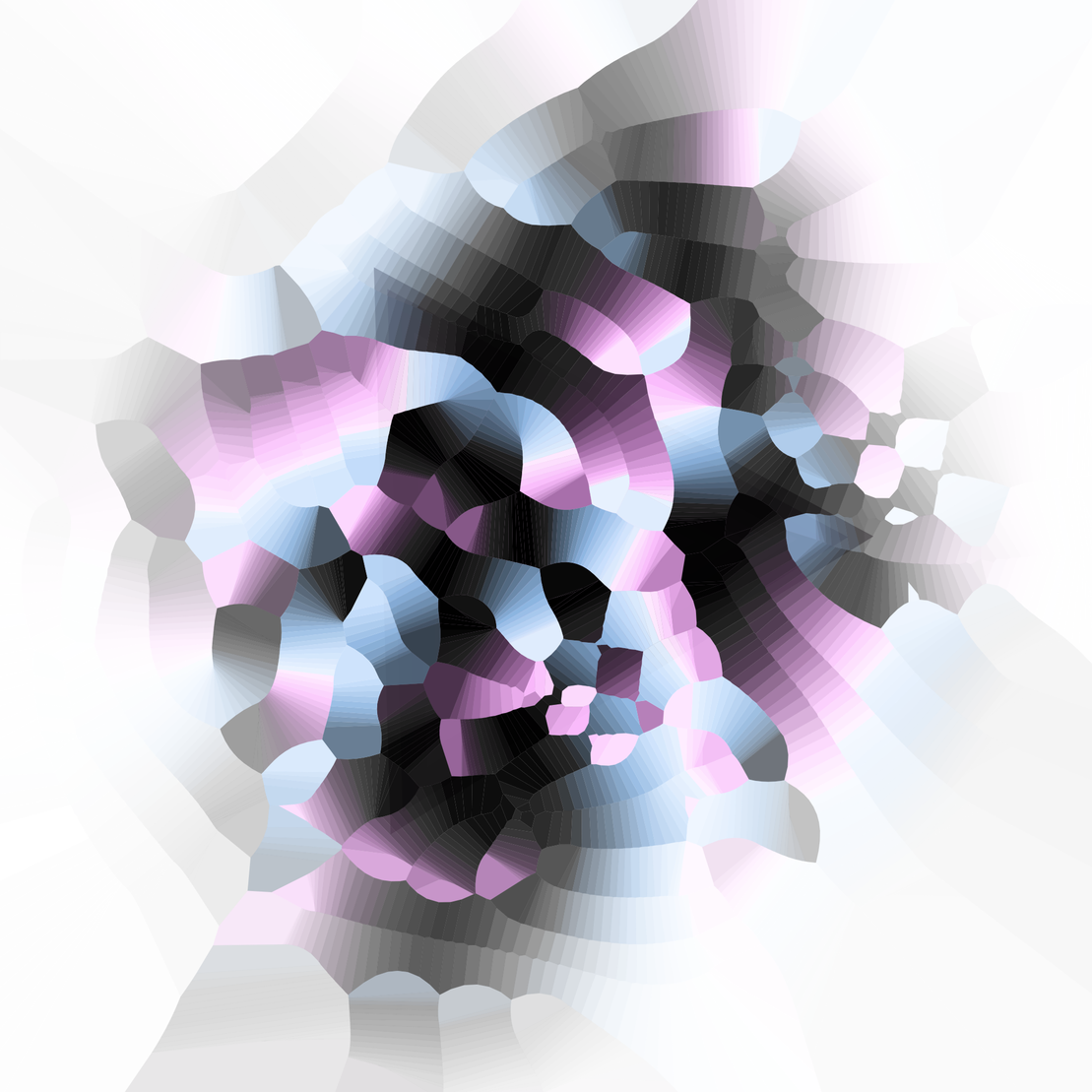
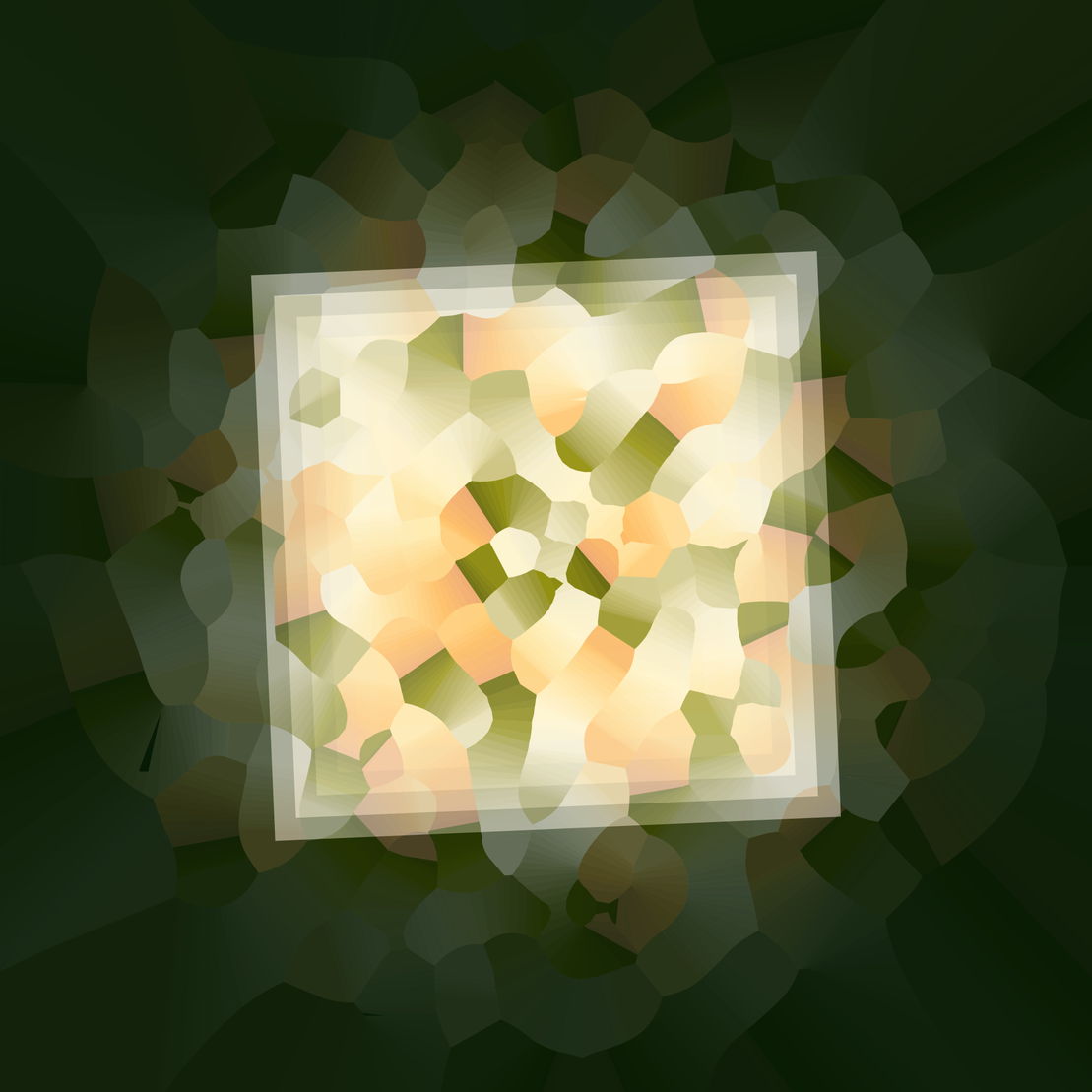
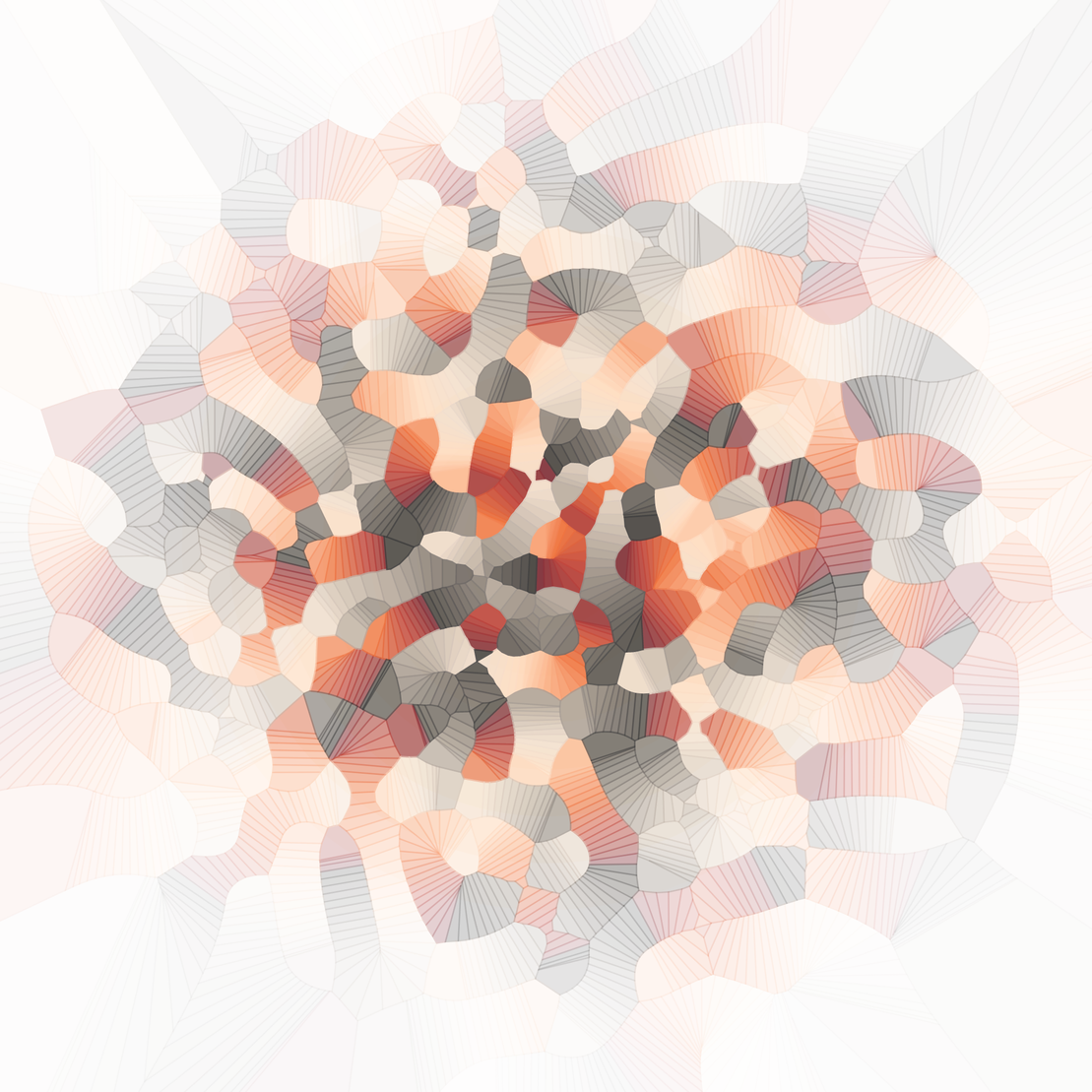
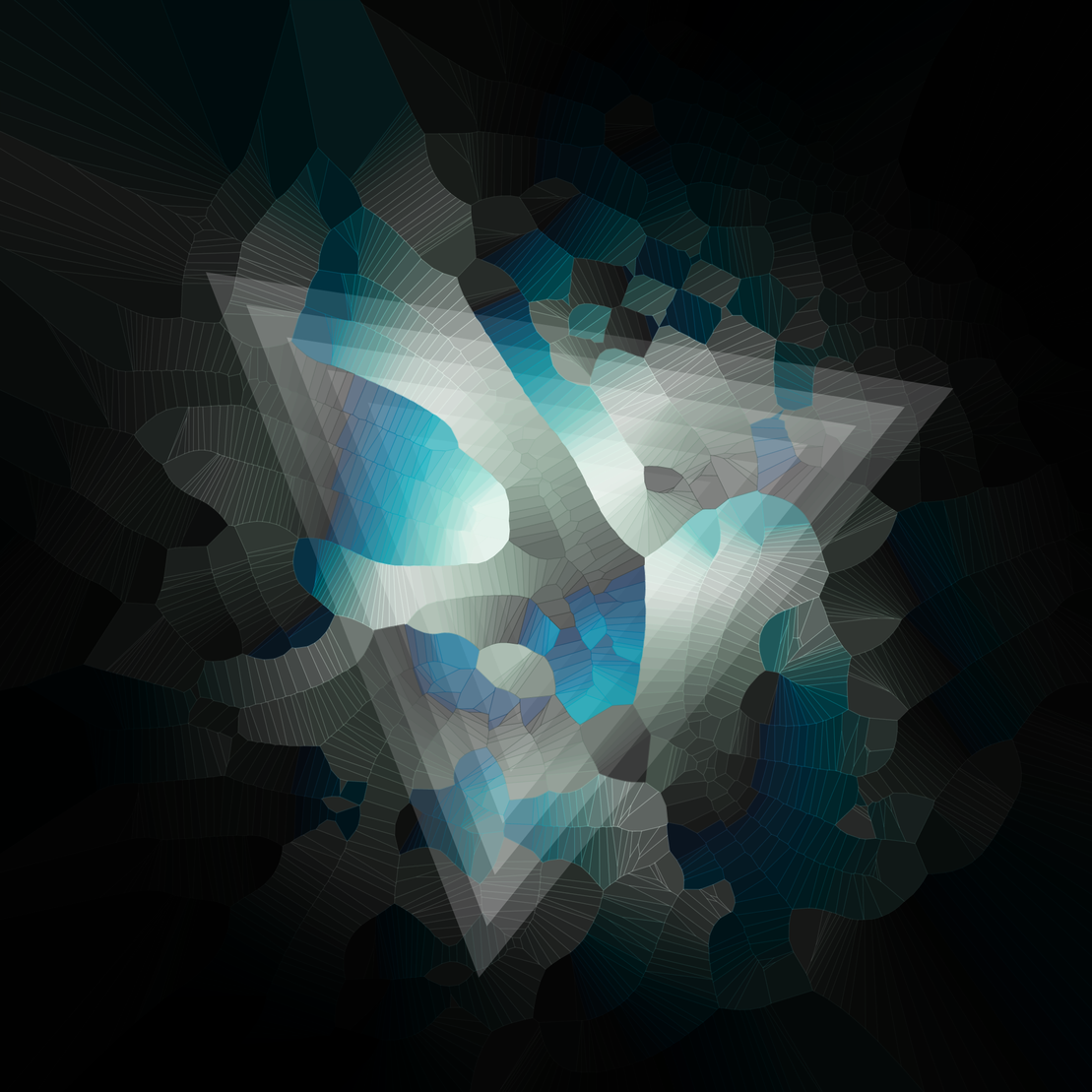
Cutting an optimization process short can lead to interesting results. This series builds on the earlier Sneronoi series but doesn't attempt to run to full convergence. Images are generated by gradient descent and the optimization process is shown as an animation. Each piece is considered final when the animation stops.

There are ten different color palettes, each with four different options for background colors. There are also different shapes drawn in the background and different modes in which the foreground and the background are blended together. In combination, these options result in a wide range of distinct visual outputs. Made with D3.

Press 's' to save as svg.

Press 'p' to save as png (4000x4000).

Author:
Claus O. Wilke
https://clauswilke.com/art
https://twitter.com/clauswilke