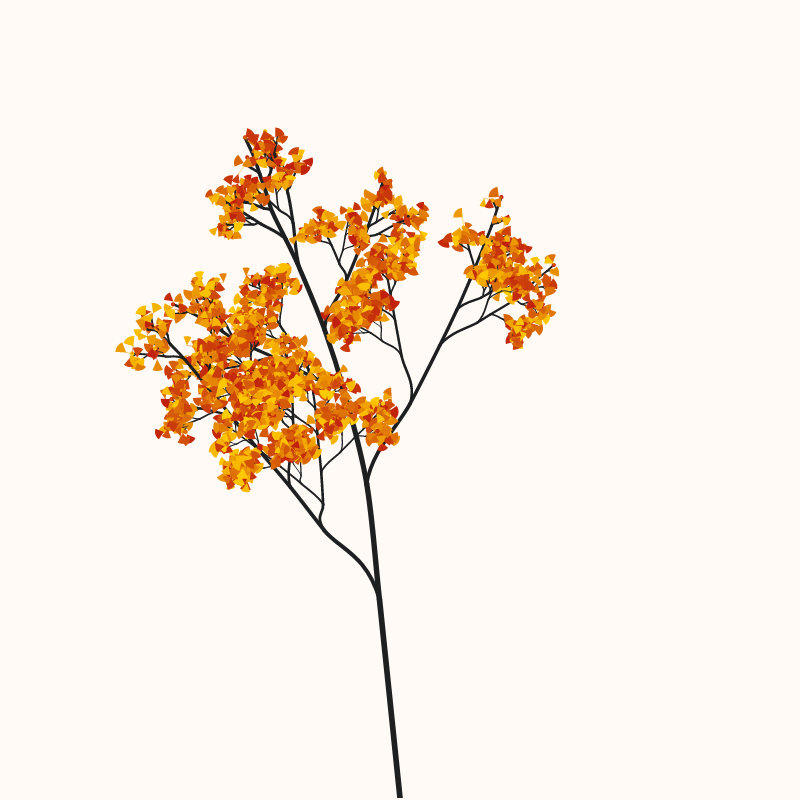
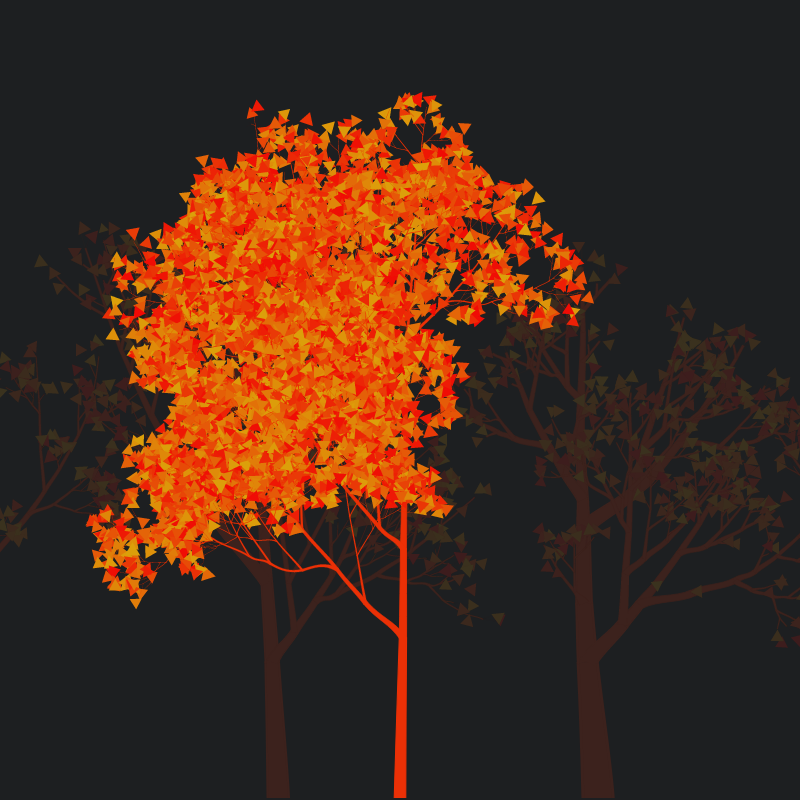
There is something almost subversive about generative art that mimics nature. Rather than excelling at abstract mathematical beauty, it tries to hold the complexity and elegance of the natural world, all while attempting to be interesting art. This makes it a different kind of challenge than the mathematical attractors and flow fields that are common across generative art.

It may be ironic then that the solution to natural forms is very often mathematical. The trees in this work are built recursively as a graph, much like how one could build a fractal. But chaos is mixed in at each step to allow the shapes to vary, and reduce their self-similarity. This is a different approach to L-systems but the results are very similar.

In this token, there are:
- Three styles of tree.
- Twelve colour schemes (six day, six night)
- Four shapes for leaves.
- Five leaf patterns
- And other entropy that cannot be enumerated.